|
Alessio Corti,
Imperial College, London
|
The LMS-MARM-NCRST
lectures on
"From the
Integrals of elementary functions to the
monodromy of the Picard-Fuchs Equations"
or "a
friendly interdisciplinary introduction to
Algebraic Geometry"
Notes
Why is so easy to
express the integral of (x3-x2)-1
in terms of elementary functions while is so
hard to find the same with (x3-x)-1?
Following the Arianna thread to solve the
enigma of this exciting yellow drama will
surprisingly provide a friendly introduction
to algebraic geometry, from a truly
interdisciplinary point of view. The course
is divided in four lectures of fifty
minutes each, as follows
Summary
- Integrals and elementary functions
- Plane algebraic curves: conics and cubics
- Elliptic integrals and parametrizations of
plane cubics
- The complete elliptic integral and the
Picard–Fuchs equation. The global monodromy
of the Picard–Fuchs equation
|
|
|
|
| Marina
Marchisio,
Università di Torino,
Italy
|
The LMS-MARM-Pupkewitz
Lectures on "Teaching and learning
Mathematics in the Digital Era"
The Covid-19 pandemic
has accelerated a process of substantial
renewal of teaching with technologies. In
this new perspective teachers were required
to make an educational investment that is
increasingly capable of innovating and
differentiating teaching strategies to make
them more suitable for the current era and
the needs of learnerss, taking advantage of
the digital skills.
The
mini-course is focused on the key role of
the Digital Learning Environments in the
teaching and learning processes of
Mathematics and other STEM disciplines,
the methodologies and theoretical
approaches that they allow to introduce
and to empower like adaptive teaching,
problem posing and solving, automatic
formative assessment, collaborative
learning and team working. The use of
Learning Analytics and Open Educational
Resources will also be discussed and
finally some examples of good practices
will be presented. During the mini-course the
participants will be invited to reflect on
the challenges of the new hybrid post-covid
scenario and will be guided in the design of
teaching activities. The course is devoted
to both students and scholars professionally
interested in mathematical training as well
as to math teachers in the High school who
wants to explore new teaching ways to be
implemented with their own learners, who
are also warmly encouraged to actively
participate to the lectures.
Summary
|
|
|
|
Sandile Motsa
University of Eswatini
|
The MARM-NARM
Lectures on "Block hybrid methods for solving
dynamical systems"
Mathematical models with applications in Engineering
and Science are best cast in differential equations
form. The differential equations that closely
approximate real-life phenomena include non-linear
dynamical systems that do not have closed-form
solutions. A myriad of numerical methods for solving
different types of non-linear dynamical systems can be
found in the literature. The choice of numerical
methods to be used depends on many factors including
the preference of the researcher, the complexity of
the problem and the nature of the differential
equation. The aim of these lecturers is to introduce
the audience to block hybrid methods that can be used
to solve basic to very complex initial value problems,
including stiff equations. The lectures give an
introduction to the development, analysis and
application of hybrid block methods in the solution of
non-linear initial value problems. Examples from
population dynamics, chemical reaction kinetics, and
fluid mechanics will be considered for numerical
experimentation using Mathematica and Matlab.
Summary
|
|
|
Luigi Preziosi, Politecnico di
Torino,
Italy |
The Politecnico di
Torino Lectures on "Mathematical modelling for
biomedical, environmental sciences and more"
The aim of the lectures is to teach how to conceive
mathematical models for specific applications stating
from the related phenomenological observation of the
phenomena involved. The basic mathematical tools will
then be explained. So, with the aim of pairing
mathematical frameworks and application the following
topics will be covered:
Summary
|
|
|
Martin Mugochi
University of Namibia
|
|
The LMS-MARM Lectures
on
"Presenting Frames"
This talk is a
3-part lecture series in which we
present frames as distributive lattices
satisfying the so-called infinite
distibutive law. On one hand frames are
viewed as Heyting algebras, on the other
as generalized lattices of “opens”. The
latter view enables one to revisit many
classical results of general topology -
an exercise dubbed as “doing topology
without points”, “pointfree topology” or
“pointless topology” - with the benefit,
sometimes, of not having to rely heavily
on choice principles.
Keywords and phrases: complete lattice,
frame, locale, sober space, spatial
locale, sublocale
|
|
|
|
Samuel Nuugulu
University of Namibia
|
The
Namibia Lectures on "Stock Markets Predictions using
Supervised Machine Learning Techniques"
Stock
markets are driven by many factors, these
factors are either based
on the fundamental or the technical aspects of
the market. The
advent of financial technology, led to the
propagation of high
volume of trading data. The veracity of such
data makes it
almost impossible
for market participants to draw any rational
investment/trading insights at face value. This
talk serves
to explore how
supervised machine learning (ML) techniques can
be used in synthesising
market news narratives, technical indicators and
actual stock
price data to draw insights on possible future
market movements. Features
such as polarity of news, aggregate sentiment, numerous
stock price information, current trend as well
as relative strength
index (RSI) were used to train the models, with
future stock trend
as the target variable. Among the fitted models,
the Gradient Boosting
Regressor model came out superior with the
highest $R^2$ and
least negative mean squared error in all three
considered stocks,
namely, Facebook, Tesla and Twitter.
|
|
|
Ursula Zich,
Politecnico di torino
|
The LMS-MARM-AngloAmerican
Lectures on "A
journey from the visual to the Geometric
thinking through Origami spectacles"
Origami is the ancient
japanese art of paper folding creating elegant
and often news geometric models. Just by
playing with paper, which will be provided by
the organization along the course, the young
audience of learners/students and possibly
professionals in mathematical teaching,
will be introduced in the marvelous world of
geometrical thinking via a visual thinking. A
model is a representation of an idea, an
object or a process and is used to describe
and analyze a transformation. Origami involves
both spatial and emotional intelligence.
Its models have tangible properties that
make them privileged laboratories for learning
and teaching geometry. The outcome of the
modeling is part of the process but not
necessarily the goal. The modeling process is
an opportunity to learn the geometric language
and see the relationships between the figures
that are created. In short, this course is a
friendly, gentle introduction to geometry
aimed to dig up the young mathematicians
hidden in the girls and boys who will attend
the lectures. We thanks the Namibia
AngloAmerican Foundation, especially
careful with the needs of young learners,
which has suggested and inspired the course.
Summary
- Physical models to visualize
geometry and learning geometric
language: why origami?
- Origami to discover geometry
- Origami to show geometry
- Geometry to design origami
|
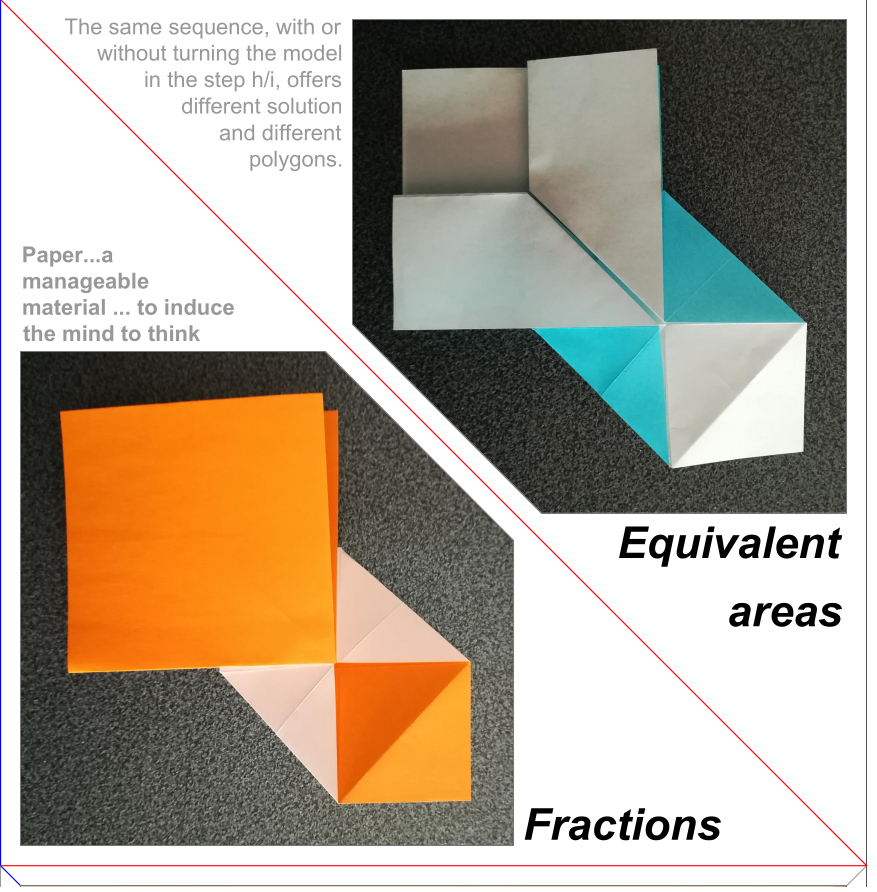
picture by Ursula Zich
|
|
|
|
Special General
Lecture by Alberto Conte, Accademia
delle Scienze, Torino (Italy)
|
|
Field Medals
|
|
This
talk is aimed for a general audience, even
of not professional mathematicians, with a
special focus to young people imaging a
future as scientists.
The Fields Medal
is the most important prize in
Mathematics. It is awarded every four
years, on the occasion of the
International Congress of Mathematicians,
to two, three or four mathematicians under
40 years of age for "Outstanding
Discoveries in Mathematics". The first
medals were awarded in 1936 and the
following continuously from 1950 up to
2022.
We will survey the
scientific work of the most important of
the 68 medallists and how it influenced
the development of Mathematics from the
middle of 20th Century up to now. |
|
